07 June,2019
Table of Contents
Find the Cartesian equation for the curve, r=cos θ
Do you recognise this equation? Of course, you do. It is the Cartesian equation. The very mention of the words “solve the Cartesian equation” evokes anxiety in nearly every student.
Students who pursue maths have to write assignments on the Cartesian equation. The problem arises when they have vague or little idea about the fundamental theories of the Cartesian equation. Comprehending the complex theories and the accurate implications of formulae baffle them the most. On asking to do a remarkable assignment on the Cartesian equation, they begin having nightmares and starts breaking into cold sweats of exasperation.
If you are struggling to understand the fundamentals of the Cartesian Equation, then reading this blog will surely help you.
Let us discuss in
Mathematician Rene Descartes discovered the concept of Cartesian Equation or Cartesian coordinates in the 17th century. It revolutionised the world of mathematics by providing the first systematic link between algebra and Euclidean geometry.
In mathematics, the Cartesian coordinate system is used significantly to determine each point in the plane through two numbers, usually called the x-coordinate and the y-coordinate of the point. To define the coordinates, two perpendicular directed lines – the ‘x-axis’ and the ‘y-axis’ is specified. These are then marked off on the two axes.
The Cartesian equation represents a locus L in the n-dimensional Euclidean Space. It takes the form: L: f (X1,….,Xn) =0,
Where, the left-hand side represents some expression of the Cartesian coordinates, x1…xn.
If solving Polar equations is making you second-guess your future dream to be a nuclear physicist, no need to worry. Almost every Pre-calculus student has faced the same daunting challenge.
To help you out, we have provided a five-step method
Step 1: Identify the form of the equation:
A quick glance at the equation should give you an idea what form it is in. If it incorporates the rs and θs, it is the form of polar equation. If it incorporates xs and ys, it is in the Cartesian or the rectangular form.
Suppose, you have to covert the equation 5r=sin (θ). You can deduce that this is a polar equation.
Step 2: State your objective:
If the equation is in the polar form, your aim will be to convert it in a way that you are left with xs and ys. If it is the form of Cartesian or rectangular, your goal will only to have rs and θs. Remind yourself of your objectives to refrain from getting stuck halfway through converting the equation.
In the example given above, your goal should be to arrive at an equation that only consists of x and y terms.
Step 3: Examine the equation:
Take a moment to examine the equation. Look for some key components in the equation. Look at the table below to get some idea.
| If the equation is in the polar form, look for…. | If the equation is the Cartesian form, look for… |
| R²= | X²+ y² |
| Rcos(θ)= | X |
| Rsin (θ)= | Y |
Figure 4: A table representing equations in Polar and Cartesian form
Looking at the equation above, you can understand that the right hand side (RHS) could turn into r sin(θ), but it is missing an r term. The left hand side (LHS) could turn into 5r2, but it is also missing an r term. Since, both the RHS and LHS misses the same term, multiply both sides by r.
5r= sin (θ)
5r2= r sin (θ)
Step 4: Substitute away:
Bear in your mind, Step 2, and start substituting.
Now, that you have an equation with terms that you can convert easily, start to substitute.
5r2= rsin (θ)
5 (x2+y2)= y
Step 5: Combine like-terms and complete squares (where required)
Simplify the equation by combining the like terms. Where you consider appropriate, mostly if you have x²s and y²s, try to complete the square. A fully simplified equation will be able to express r in terms of θ or y in terms of x.
Finally, you can combine the terms and simplify the equation. To get the accurate result, some mathematicians will ask you to set the RHS to zero if the equation is truly simplified. Others will ask that if you factor out any recurring term. All three equations given below are in varying degrees of simplification.
5 (x2+y2) = y
5×2+ 5y2-y =0
5×2+y (5y-1)= 0
Hence, the equation is converted from Polar to Cartesian in simple five steps. If you face difficulties, you can take the help of Polar Equation to Cartesian Equation Calculators available online.
Most students are often asked to “eliminate the parameter to find a Cartesian equation of the curve”.
Let’s find out how you can convert parametric to
Consider the following:
x=e4t, y= t+9
1. Eliminate parameter to find the Cartesian equation of the curve.
2. Sketch a curve. Indicate with an arrow the direction in which the curve is traced as the parameter T increases.
Step 1: So, as you see x= e4t
You have to get rid of the parameter t and solve t from the y equation. So,
Y= t+9
y-9=t
x= e 4(y-9)
It can be simplified further, or it can be said,
Y=t+9
X= e4t
Take the natural log of both sides, and divide by 4. So,
lnx= 4t
lLnx/4= t
Plug t back into the y equation,
y=ln(x)/4 +9
Now both of these equations are correct. They are both in terms of Cartesian coordinates. Normally the equation set equal to Y is more acceptable. Typically, it is the traditional method in which equations are written.
Like, y= mx+b
y is typically the isolated variable in the equation. Therefore, the set equation of
y=ln(x)/4 +9 is more acceptable than x= e 4(y-9).
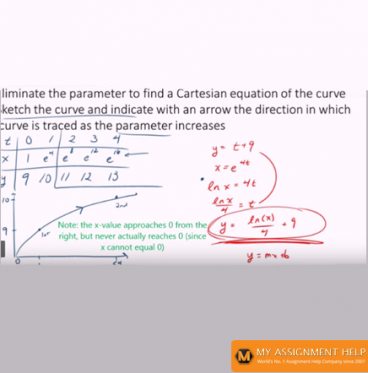
Step 2: Now sketch the curve as the parameter T increases. Set up a table using three variables t, x, and y. This is slightly difficult as there are no limitations on T. You know that there is an exponential function, and X will not be less than 0. Look at the table to get an idea.
| T | 0 1 2 3 4 |
| X | 1 e4 e8 e12 e16 |
| Y | 9 10 11 12 13 |
Figure 6: A table representing the values of t, x, and y
Here, t is independent variable. x and y are dependent variables.
Now, draw a graph. It will look something like this.
Following these steps, you will able to convert parametric into Cartesian equation remarkably without taking the help of a Parametric equation calculator.
We have provided the simplest way to find the parametric equation of a parabola.
Suppose, you are asked to find the parametric equations of the parabola
(y-2) ² = 8 (x-2)
Therefore, the required solution will be:
The given equation (y-2) ²= 8 (x-2) is of form of (y-k) ²= 4a (x-h). On comparing equation (y-2) ²= 8 (x-2) with equation (y-k) ²= 4a (x-h).
You will get,
4 a=8 ⇒ a=2, h=2 and k=2.
Therefore, the parametric equations of the given parabola are x= 2t²+ 2 and y= 4t +2.
Finding the solutions to the Cartesian equation of a curve often becomes somewhat tricky and challenging for the students. Therefore, they take the help of Cartesian equation calculators available online to be relieved of their drudgery.
Therefore, we have provided a simple method to find the Cartesian equation of a curve as remarkably and efficiently as the Cartesian equation calculator.
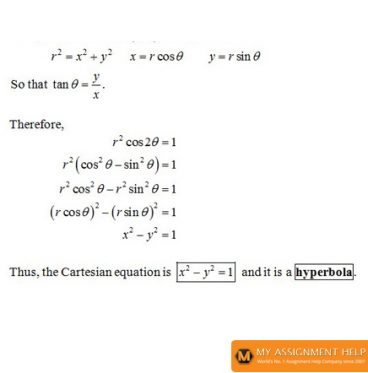
Suppose you are required to find the solution of this Cartesian equation:
X= 2+t² y= 4t
You notice that the equation is a curve equation.
To find the solution accurately, you are required to solve parametric equations simultaneously:
If y= 4t, then divide both sides by 4 to find (1/4)y =t.
The newly found value of t can be substituted in the equation for x:
X= 2+ (1/4(y)) ² – expand bracket (square both ¼ and y) in order to derive
X= 2+ 1/16y²
The final equation is already in the form of Cartesian. It includes variables x and y. However, you have to rearrange the equation to find the standard ‘y’= form:
Therefore,
X= 2+1/ 16 y² (From both sides subtract 2)
x-2= 1/16 y² ( By 16 multiply each side)
16 x-32 = y² (Take the square roots of both sides)
Y= SQRT (16x-32)
A level curve of a function is a curve of points, where the function incorporates constant values. The level curve is simply a cross section of the graph of equation. When it is equated to some constant values, like a function of two variables say x and y, then you can find the curve of points (x, y), where the function has a constant value.
Let us have a look at the example given below to understand how you can find the solution of a level curve equation without the use of level curve calculator.
Suppose, you are asked to find the level curves of heights C= 0, 1 for f (x,y)= x+y+1
Therefore, the solution will be,
For height C= 0
x+y+1= 0
y= -1-x
For height C=1
x+y+1=1
y= -x
For these two values of y, you can draw the curves. For C=0 you will have a straight line as the standard equation of the line is y= mx+c.
On comparing, you will achieve the result
Slope m= -1 and the intercept is -1.
Therefore, you will get a line, and similarly for c=1 you can draw a line with slope-1 and intercept 0.
Wrapping it up
While assignments on Cartesian equation can give you a challenging time, this blog can be just a solution to it all. With this comprehensive guide to all things on Cartesian equations, you can nail that assignment like never before. Save this to bookmark tab on your laptop so you can trace it easily while solving the complicated equations. You can also take a few pointers on drawing up a slant for that calculus assignment.
If writing a tricky and complicated maths assignment is scaring you, then get in touch with the math experts of MyAssignmenthelp.com right away.
Are you unable to figure out how to solve the complicated maths equations? Fret not. We, at MyAssignmenthelp, boast of a pool of brilliant experts. They are highly qualified and have decades of experience. The qualified professionals can help you with a diverse range of topics on trigonometry, calculus, geometry, or algebra.
Our supreme quality services are available at the most jaw-dropping deals that will surely not burn a hole in your pocket. To hire our services, call us or drop an email at [email protected], and we will reach back to you in no time.
Hi, I am Ethan, a nerd by nature, a data scientist by profession and, a gadget lover at heart. Thanks for stopping by and sparing a few minutes to know me. I would like to let you know, apart from being a Maths lover and a tech freak I have a fetish for English writing. I am into everything that spells maths, technology, and essays. I have an experience of 10 years as a data scientist and that of 5 years as a freelance English essay writer at myassignmenthelp.com. I like sharing my experiences with people, which further makes me an occasional blogger.
WhatsApp
